For a module at an arbitrary tilt and orientation, the equation becomes a little more complicated:
Solar Intensity
α is the sun elevation angle, and Θ is the sun azimuth angle. β is the module tilt angle. A module lying flat on the ground has β =0°, and a vertical module has a β =90°. Ψ is the azimuth angle that the module faces. The vast majority of modules are aligned to face towards the equator. A module in the southern hemisphere will be facing north with Ψ = 0° and a module in the northern hemisphere will typically face directly south with Ψ = 180°. Smodule and Sincident are respectively the light intensities on the module and of the incoming light in W/m², the Sincident being a direct only component.
A module that directly faces the sun so that the incoming rays are perpendicular to the module surface has the module tilt equal to the sun's zenith angle (90 - α = β), and the module azimuth angle equal to the sun's azimuth angle (Ψ = Θ).
The following calculations combine the sun's position with the Airmass formula and then calculate the intensity of light incident on a module with arbitrary tilt and orientation.
Light Intensity
Using Vectors to Calculate Solar Direction
As the number of tilts and orientations become more complicated, it is often easier to convert the solar directions of azimuth and elevation to vectors. An example is a tilted module on a building that is also at an arbitrary tilt and orientation. The simplicity of using vector comes from the fact that the reduction in intensity of light on a tilted surface is simply the dot product between the incident ray and the normal to the module.
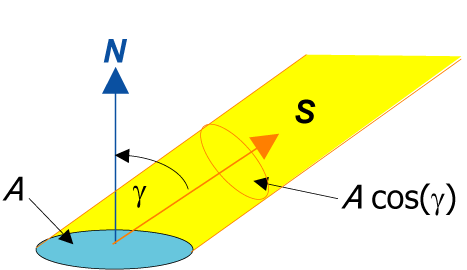
Light striking a surface at an angle is spread out over a larger area. The reduction in intensity is the dot product of the unit vectors S and N
Solar Intensity Vector
where Smodule and Sincident are as defined before, S is the unit vector point towards the sun, and N is the unit vector normal to the surface of the module. γ is the angle between the two vectors
