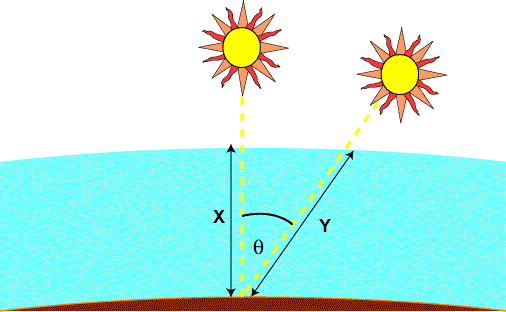
The Air Mass is the path length which light takes through the atmosphere normalized to the shortest possible path length (that is, when the sun is directly overhead). The Air Mass quantifies the reduction in the power of light as it passes through the atmosphere and is absorbed by air and dust. The Air Mass is defined as:
Air Mass Zenith
where θ is the angle from the vertical (zenith angle). When the sun is directly overhead, the Air Mass is 1.
Air Mass Zenith
A more detailed model showing the effect of airmass on the solar spectrum is available at the PV Lighthouse Solar Spectrum Calculator.
An easy method to determine the air mass is from the shadow of a vertical pole.
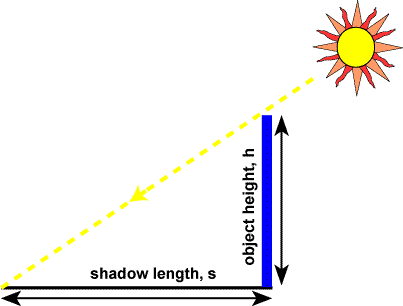
Air mass is the length of the hypotenuse divided by the object height h, and from Pythagoras's theorem we get:
Air Mass Shadow
Air Mass Shadow
The above calculation for air mass assumes that the atmosphere is a flat horizontal layer, but because of the curvature of the atmosphere, the air mass is not quite equal to the atmospheric path length when the sun is close to the horizon. At sunrise, the angle of the sun from the vertical position is 90° and the air mass is infinite, whereas the path length clearly is not. An equation which incorporates the curvature of the earth is1:
Air Mass Curvature
Standardised Solar Spectrum and Solar Irradiation
The efficiency of a solar cell is sensitive to variations in both the power and the spectrum of the incident light. To facilitate an accurate comparison between solar cells measured at different times and locations, a standard spectrum and power density has been defined for both radiation outside the Earth's atmosphere and at the Earth's surface.
The standard spectrum at the Earth's surface is called AM1.5G, (the G stands for global and includes both direct and diffuse radiation) or AM1.5D (which includes direct radiation only). The intensity of AM1.5D radiation can be approximated by reducing the AM0 spectrum by 28% (18% due to absorption and 10% to scattering). The global spectrum is 10% higher than the direct spectrum. These calculations give approximately 970 W/m2 for AM1.5G. However, the standard AM1.5G spectrum has been normalized to give 1kW/m2 due to the convenience of the round number and the fact that there are inherently variations in incident solar radiation. The standard spectrum is listed in the Appendix page.
The standard spectrum outside the Earth's atmosphere is called AM0, because at no stage does the light pass through the atmosphere. This spectrum is typically used to predict the expected performance of cells in space.
Intensity Calculations Based on the Air Mass
The intensity of the direct component of sunlight throughout each day can be determined as a function of air mass from the experimentally determined equation 2:
Intensity Sea Level
where ID is the intensity on a plane perpendicular to the sun's rays in units of kW/m2 and AM is the air mass. The value of 1.353 kW/m2 is the solar constant and the number 0.7 arises from the fact that about 70% of the radiation incident on the atmosphere is transmitted to the Earth. The extra power term of 0.678 is an empirical fit to the observed data and takes into account the non-uniformities in the atmospheric layers.
Sunlight intensity increases with the height above sea level. The spectral content of sunlight also changes making the sky 'bluer' on high mountains. Much of the southwest of the United States is two kilometers above sea level, adding significantly to solar isolation. A simple empirical fit to observed data 3 and accurate to a few kilometers above sea level is given by:
Intensity
where a = 0.14 and h is the location height above sea level in kilometers.
Even on a clear day, the diffuse radiation is still about 10% of the direct component. Thus on a clear day the global irradiance on a module perpendicular to the sun's rays is:
Global Irradiance
Radiation Intensity
- 1. , “Revised optical air mass tables and approximation formula”, Applied Optics, vol. 28, pp. 4735–4738, 1989.
- 2. , Applied Solar Energy. Addison Wesley Publishing Co., 1976.
- 3. , “The measurement of solar spectral irradiance at different terrestrial elevations”, Solar Energy, vol. 13, pp. 43 - 50, IN1-IN4, 51-57, 1970.
- Log in or register to post comments
- 5 comment(s)
 Español
Español 한국어
한국어 简体中文
简体中文 Bahasa Indonesia
Bahasa Indonesia